Assignment #7: Tangent Circles
by
Laura Singletary
This investigation begins with the following problem:
Given two circles and a point on one of the circles. Construct a circle tangent to the two circles with one point of tangency being the designated point.
To begin, we must construct two circles: the circle centered at A and the circle centered at B. It is important to consider the possibilities of the placement of the circles, for there are three cases: Case 1: one circle is located "inside" the other circle, Case 2: the circles are intersecting therefore sharing two common points, Case 3: or the circles are disjoint and share no interior points. Evaluate the three different cases below and consider how they might effect constructing a circle tangent to the two circles with one point of tangency being the designated point.

Consider Case 1, where one circle is located "inside" the other circle.There are two possible solutions for constructing the tangent circle to the circles centered at A and B with a designated point of tangency. To explore, open the GSP files for the first solution and the second solution.
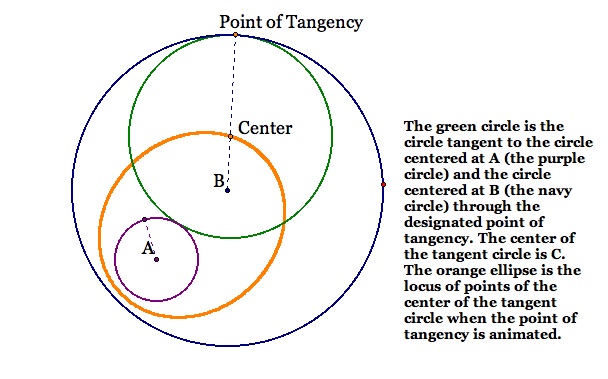
Below we find the construction of the tangent (green) circle for Case 1, call it the first solution for Case 1:
Below is a diagram highlighting the locus of points of the center of the tangent (green) circle when the point of tangency is animated. For the first solution for Case 1, we notice that the Locus of the Centers of the tangent circle seems to be an ellipse.
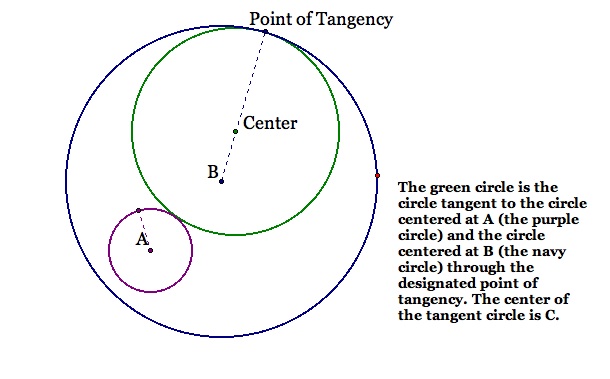
Below we find a different construction of the tangent (green) circle for Case 1, call it the second solution for Case 1:
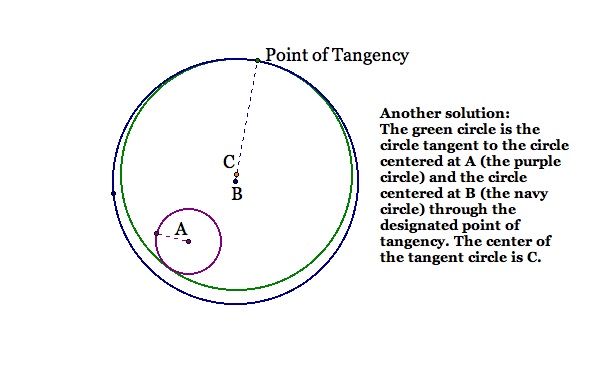
Below is a diagram highlighting the Locus of the points of the center of the tangent (green) circle when the point of tangency is animated. For the second solution for Case 1, we notice the Locus of the Centers of the tangent circle seems to be an ellipse.

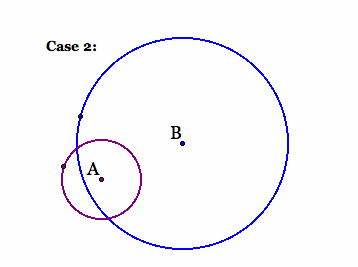
Consider Case 2, where the circles are intersecting and are therefore sharing common points. There are two possible solutions for constructing the circle tangent to the circle centered at A and the circle centered at B passing through the designated point of tangency. To explore, open the GSP files for the first solution and the second solution for Case 2.
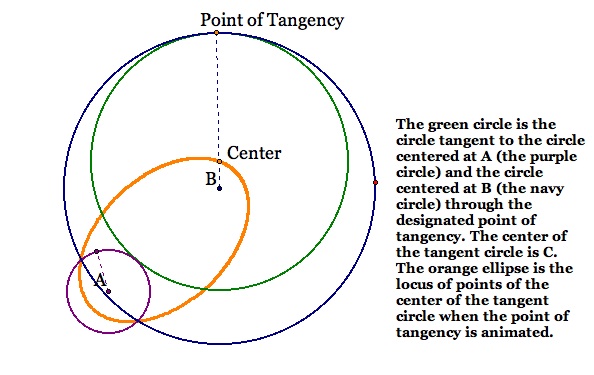
Below we find the construction of the tangent (green) circle for Case 2, call it the first solution for Case 2:

Below is a diagram highlighting the locus of points of the center of the tangent (green) circle when the point of tangency is animated. For the first solution for Case 2, we notice the Locus of the Centers of the tangent circle seems to be an ellipse.
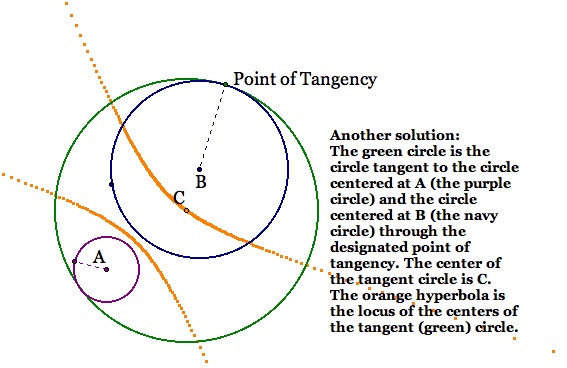
Below we find the construction of the tangent (green) circle for Case 2, call it the second solution for Case 2:
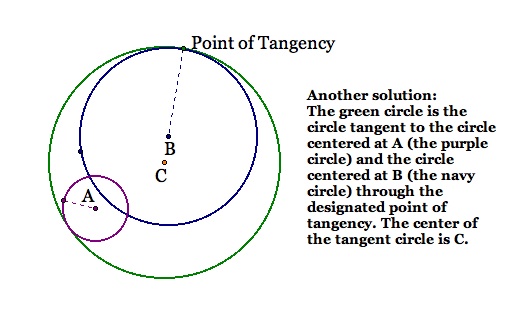
Below is a diagram highlighting the locus of points of the center of the tangent (green) circle when the point of tangency is animated. For the second solution for Case 2, we notice that the Locus of the Centers of the tangent circle is a hyperbola.
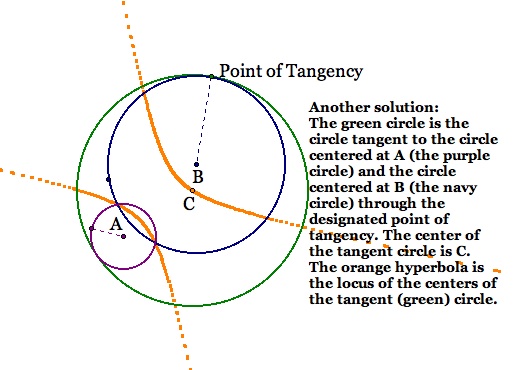
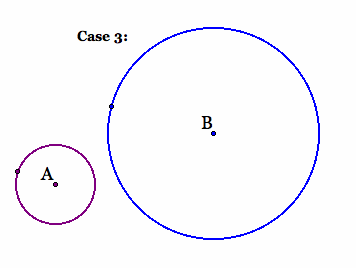
Consider Case 3, where the circles are disjoint and share no interior points. There are two possible solutions for constructing the circle tangent to the circle centered at A and the circle centered at B passing through the designated point of tangency. To explore, open the GSP files for the first solution and the second solution for the 3rd Case.
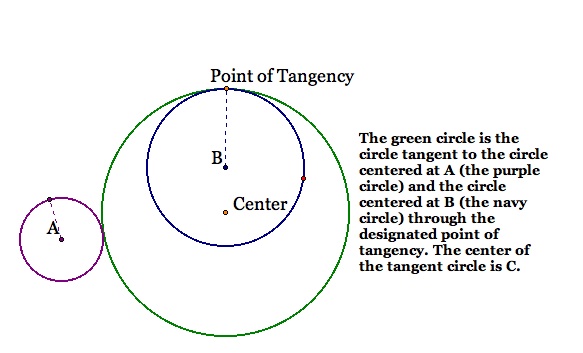
Below we find the construction of the tangent (green) circle for Case 3, call it the first solution for Case 3:
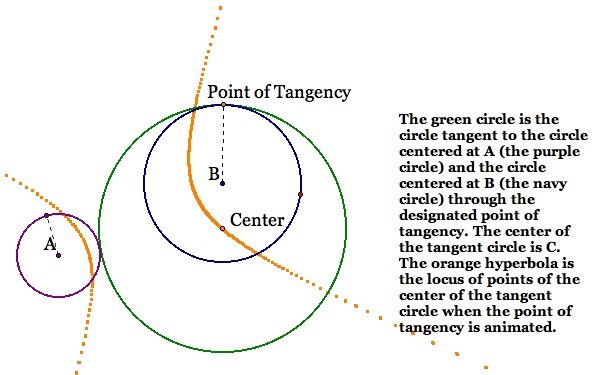
Below is a diagram highlighting the locus of points of the center of the tangent (green) circle when the point of tangency is animated. For the first solution for Case 3, we notice the Locus of the Centers of the tangent circle is a hyperbola.
Below we find the construction of the tangent (green) circle for Case 3, call it the second solution for Case 3:
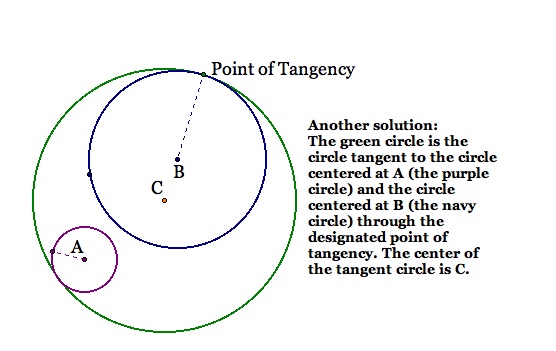
Below is a diagram highlighting the locus of points of the center of the tangent (green) circle when the point of tangency is animated. For the second solution for Case 3, we notice the Locus of the Centers of the tangent circle is a hyperbola.